Suppose you're playing the standard Monty Hall problem, but there are 6 doors and you get 5 guesses. You make your original 5 guesses, then Monty Hall opens 4 doors from your original choices and shows them to be wrong, leaving your 5th guess a mystery. There is 1 other door you haven't chosen yet. Monty Hall tells you that you have spent 4 of your 5 guesses since he gave you this extra info for free and you only have 1 now. You can choose to stay on your currently selected door, or you can change to the unselected one. Do you switch?

Taking this to the ridiculous extreme, suppose you got 99 guesses originally out of 100 doors, and Monty Hall exposes 98 of your original selection to be wrong. You can keep your last door or move to the door you didn't select.

Mathematically speaking, I almost certainly chose the right door somewhere in my original 99 guesses of my second comment setup as opposed to the 1% chance I chose every wrong door on my first attempt. I am compelled to take the 99% chance certainty over the 1% chance and keep my original pick that might be right.

We can apply similar logic to the main question of this poll.

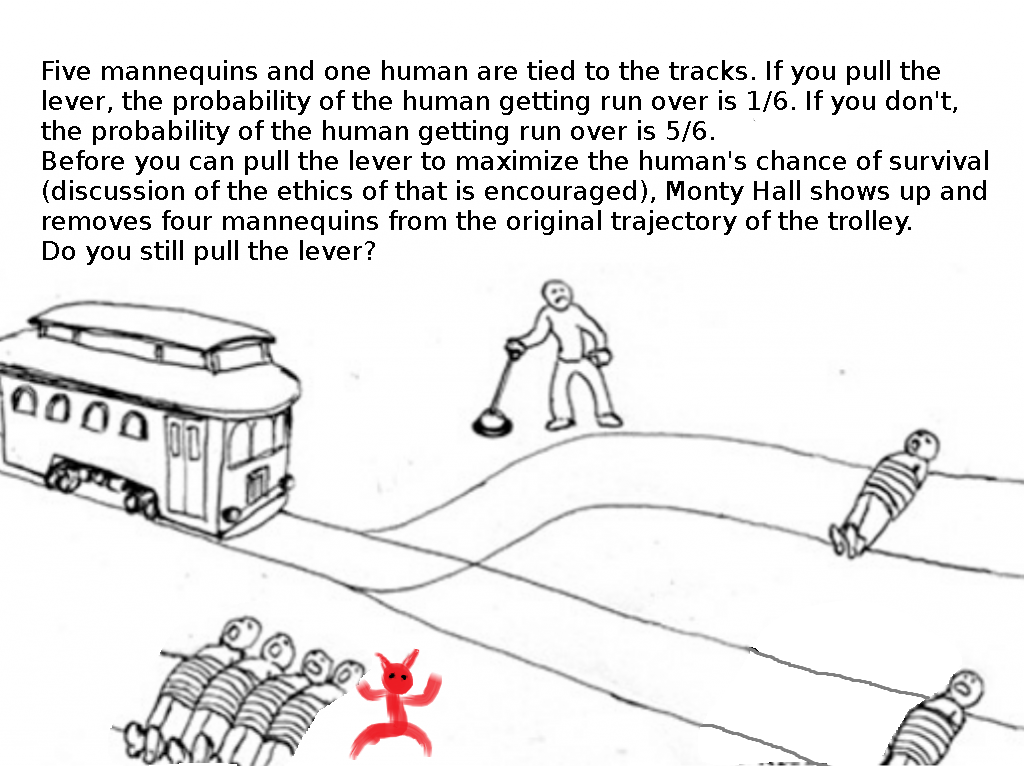
There was a 5/6 chance of the human being on the 5 body track. No new information is unlocked when Monty reveals his information since we are reducing our 'amount of correct guesses' we have to work with. It's still a 5/6 chance.

Flip the switch. Run over the 1 body track instead of the original 5 body track, assuming utilitarianism trumps deontology.

Just to really cement the analogy I'm making, we ask ourselves if the answer to this problem changes if there were 99 bodies on the bottom track, then Monty reveals 98 to be mannequins, and 1 body remains on the top track and bottom track after that. Do you flip the switch?

even if you get stuck on this one bc both options feel weirdly viable, you pull the lever bc 1 track gives the human either a 50/50 chance or a 5/6 chance of death and the other gives the human a 50/50 chance or a 1/6 chance of death :B

but it does make a difference whether you model this as "one person was tied to a track and then 5 mannequins were added" or "6 figures were tied to tracks and one of them is a mannequin." i think. i forgot. this hurts my brain. but "pull" is correct

Yeah I'm pretty sure this one actually is a 50/50? Hall is revealing the same 4 mannequins, all on the 5-track, no matter where the human is.

@gaming What do you mean by this? The probability is definitely not 50-50, unless you assume there was some chance that Hall would remove a mannequin from the upper track, but you are meant to assume that that is not the case.

@PlasmaBallin (I am very tired right now, take this with a pound of salt). Does this matter? In the original problem, the doors Hall reveals is dependent on your choice and the location of the car. Here, you don't have a choice, and the location of the human doesn't matter; he always reveals exactly 4 mannequins from the 5-track. There always are 4 mannequins on the 5-track.

@gaming The Monty Hall problem would still have the same solution even if you didn't get to choose the door that starts out as your guess, as long as that door is chosen in a way that's independent of the location of the car. In this problem, you're meant to assume that the human is equally likely to be in any of the six positions, so the top track takes the role of the initially chosen door.

What does matter to the solution of the Monty Hall problem is the behavior of the host. In this version, you're meant to assume that Monty Hall only removes mannequins from the bottom track, that he never removes the human, and that he always removes exactly four. This is exactly analogous to Monty Hall always opening exactly one door, which is never the one you chose initially or the one with a car behind it, in the original problem.

All things being equal deontology > utilitarianism!

@rocknrollfinance But all things are not equal. If you pull the lever, there's a 1/6 chance of killing someone, and if you don't, there's a 5/6 chance of killing someone.

@PlasmaBallin Yes, but surely it’s 1/2 either way once the mannequins go!

@rocknrollfinance No, the probability is still the same afterwards. Removing the mannequins does nothing to change it. It's the same as the Monty Hall problem.

What a strange question 😭
So before dear Monsieur Hall shows up, pulling the lever means 1/6 chance of each target being run over, and not pulling the lever means 5/6 chance it's the human and 1/30 chance it's each of the 'quins?

@gaming Five bodies are on the current track. One body is on the alternate track. Of the six bodies, five are mannequins and one is a human, but you know not which. If you do nothing, five bodies will be run over. If you pull the lever, one body will be run over. Before you can make a choice, Monty Hall shows up and removes four mannequins from the current track. Now there is one body on the current track and one body on the alternate track. Should you pull the lever or not?